If there is going to be a theme in this series of essays, it will be this, it’s essential to understand things deeply, and it is better to not know something than to understand it shallowly. For game theory, it’s easy to state its precepts; difficult to explain its limits. And the field does have limits. Our very existence helps highlight them and acts to disprove the theory’s universal applicability.
A mathematical treatment of game theory is beyond this post. However, if you’d still like to truly understand game theory, I would recommend buying a copy of Dr. Martin Osborne’s excellent text, “An Introduction to Game Theory.1” It requires minimal mathematical understanding, and if you’re studying with the solution manual2, you can gain a somewhat fluent beginner’s understanding of game theory.
Game theory, as we know it, was first formalized when, arguably, the smartest human being to ever walk the Earth realized that he was terrible at poker.
Having mastered calculus by the age of 8, moved the great mathematician Gábor Szegő to tears over his mathematical brilliance by the age of 15, young John von Neumann certainly had the intellectual pre-requisites to be brilliant at poker. He intimately understood the probabilities involved and — for a mind capable of dividing 8 digits in his head almost instantly — could keep track of the branching possibilities as the deck (or decks) played out. And yet he lost. Frequently.
Von Neumann quickly realized that no one had formalized the mathematical process of betting itself. And so, he set out to prove the minimax theorem, which formed the cornerstone of game theory and provided the mathematical basis for the entire field. He published his work in the journal Mathematische Annalenin the paper, “Zur Theorie der Gesellschaftsspiele” in 19283.
He later went on to write and publish the foundational text in the field, Theory of Games and Economic Behavior, with Oskar Morgenstern in 1944. Around the same time, he was responsible for helping build the first nuclear bombs. These two events are intertwined in the theory’s beginnings and form the seeds of our world. For if game theory had been conceived on the poker table, it was born at Los Alamos.
It is difficult to overstate the contributions of von Neumann to American strategic policy and the stability of the post-war years. Contributions that were guided by his unique abilities and framed with game theory.
He arrived at that point due to his work during the war years. In more ways than one, the nuclear standoff that our world is perpetually engaged in was created in his mind. He was the one who practically conceived of and demonstrated that the explosive lens-type device used at Trinity and Nagasaki was more efficient than the gun-type device used at Hiroshima. It required extensive calculations of the physics and mechanics of blast waves that seemed beyond the technology of the day. Until von Neumann.
A more ordinary person had left the problem as intractable, causing Oppenheimer to bring in the one person he thought could solve this seemingly impossible problem4,
“We are in what can only be described as a desperate need of your help. We have a good many theoretical people working here, but I think that if your usual shrewdness is a guide to you about the probable nature of our problems you will see why even this staff is in some respects critically inadequate…I would like you to come as a permanent, and let me assure you, honored member of our staff. A visit will give you a better idea of this somewhat Buck Rogers project than any amount of correspondence.”
When Turing was working with the Bombe to break the enigma, von Neumann was running competitions between electromechanical and electronic computers to build the bomb. The first consequential program the Harvard Mark I ever ran was for his explosive lens problem5. The electromechanical computer won, but von Neumann wrote6,
The Los Alamos group completed its work in a much shorter time than the Cambridge group. However, the punched-card machine operation computed values to six decimal places, whereas the Mark I computed values to eighteen decimal places. Additionally, Mark I integrated the partial differential equation at a much smaller interval size [or smaller mesh] and so...achieved far greater precision.
Seizing the importance of this moment, he realized that the most important work he could do, far more consequential than bombs, was computers. His ‘First Draft of a Report on the EDVAC7’ was so widely circulated that the architecture he described within it came to be known (controversially) as the von Neumann architecture, the very same architecture your devices use today.
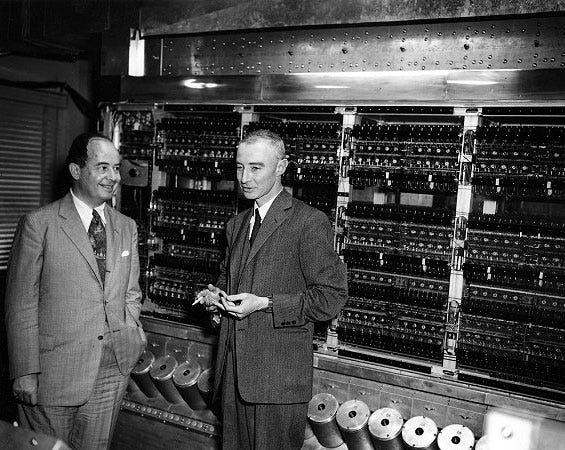
In doing so, he augured the creation of the Teller-Ulam design — he did the math to demonstrate that it was feasible and possible to create. Executed, of course, on the first digital computers. He pushed for more research into computation and drove the creation of faster computers. At around the same time, he convinced the US Government to give resources to the US Army Ballistic Research Laboratory (Wernher von Braun’s group) and the Naval Research Laboratory to pursue the development of ICBMs. He felt that multiple groups, collaborating and competing against each other, would reach the goal faster than a single group trying to build it alone8. The Top Secret committee on ICBM design would, naturally, meet in his living room.
In the post-war years, until his death by cancer, John von Neumann was, by and large, the intellect behind US defense policy. His centrality must be understood to punctuate and grasp the failure and limits of game theory. From Marina von Neumann Whitman’s “The Martian’s Daughter,”
In September of 1956, I was sitting in the anteroom of an elegant hospital suite at Walter Reed Army Hospital in Bethesda, Maryland, in a VIP wing reserved for the president and other high-ranking individuals, both civilian and military. [...] The more important consideration, though, was national security. Given the top secret nature of my father’s involvements, absolute privacy was essential [...]
Vince Ford, an Air Force colonel who had been closely involved in the supersecret development of an intercontinental ballistic missile (ICBM) [...] was assigned as his full-time aide. Eight airmen, all with top secret clearance, rotated around the clock. Their job was both to attend to my father’s everyday needs and, in the later stages of his illness, to assure that, affected by medication or the advancing cancer, he did not inadvertently blurt out military secrets.
You may have already arrived at his most famous contribution to American national security. One shaped entirely by game theory. Mutually Assured Destruction. It’s called MAD because he thought it was funny. This game theoretic policy has led the US, and the rest of the world, to spend trillions of dollars over decades to perpetuate the largest “game” ever played.
However, the implications of MAD aren’t what most people expect. Our current interpretation is a mutation of the original premise, as von Neumann examined the situation mathematically and arrived at a two-party version of Nash’s Equilibrium (John Nash generalized it to n-parties).
More precisely, nuclear annihilation is not an iterated dilemma; therefore, it is completely logical for a first strike to be the best strategic option in this scenario. If State A destroys State B and removes any capacity for State B to strike A, then State A is able to guarantee its future existence and “wins” the game.
The policy of nuclear retaliation, or a second strike, is an attempt to change the payoff function of this game and add a cost to this form of defection. It adds one more iteration to the game where the player is punished for their defection/first strike. Thereby making sure that the win-state is not attained.
However, this rationale/version of MAD starts to break down the longer that you look at it. What incentive do the submarine commanders have to launch, given that the homeland that they’ve received their orders from has already been destroyed? It is not a rational policy from the survivor’s standpoint to destroy what is left of the world and end their own existence in the process. Therefore they have an incentive to not carry out the second strike, resurrecting the first-strike problem once again.
From this perspective, and from the technology that existed in 1950, game theory predicts only one solution — strike first; strike hard. As he famously put it9,
“If you say why not bomb [the Soviets] tomorrow, I say, why not today? If you say today at five o’clock, I say why not one o’clock?”
It was, and is, a single iteration game with two players operating under a clear payoff function. Clearly, the only correct, rational choice was to annihilate rather than be annihilated.
And yet, we’re still here. Despite many, many, many close calls. Including the times when the US accidentally, almost, nuked the Carolinas. Twice. (that we know of.)
Why?

In 1962, the Soviet submarine B-59 thought that nuclear war had already broken out. They had been out of contact with Moscow for days. US Navy destroyers were dropping signaling depth charges onto them despite their presence in international waters. The captain decided to launch a nuclear tipped torpedo, an act that would have led to the start of a nuclear war.
According to Soviet Nuclear Command & Control procedure, he had to get approval from both the Soviet political officer, and the Flotilla’s Chief of Staff, who happened to be on board. The political officer said yes. Only Vasily Arkhipov, the Chief of Staff, said no. An argument broke out, but Arkhipov stood his ground. And, in doing so, he saved the world.10
Every time we have come close to a strike or a launch, there has been someone like Vasily Arkhipov in the room who has said no. When the cards have been down, people have refused to be the ones to sentence humanity to a, possibly, species-ending self-genocide.
President Nixon used to get drunk. Extremely drunk. And he would sometimes order nuclear strikes. Kissinger would usher him to bed and would get the forces to stand down. It is not unreasonable to say that one of the reasons why Nixon was hastened out of power was because of these incidents.
From Senator Sam Ervin, who led the charge in Watergate (emphasis mine)11;
Senator Sam Ervin discussed just such a possibility with majority leader Mike Mansfield before they decided on the Watergate probe. It had been, even then, “that thing which was the main fear and therefore the prime issue. Which wasn’t whether or not Nixon was a crook. Millions had been talking on both sides of that issue for more than a quarter century now. Everyone knew what the prime issue was. A certain thumb moving awkwardly towards a certain red button, a certain question of sanity... Query: if the man who holds the thumb over the button is mad...”
This question of sanity caused the probe to become a bipartisan effort. No one wants to be the one to end the world.
In Israel, during the Yom Kippur war, prime minister Golda Meir authorized the arming of nuclear warheads to strike Cairo and Damascus as a last resort. But, ultimately, she stood down and halted all military action, preventing further escalation.
All told, there have been 16 “close calls.” That we know of. The world has faced nuclear annihilation 16 times, and all 16 times, multiple people have refused to press the button despite (seeming) orders and procedures to the contrary.
Their actions are not rational. These outcomes fly against the predictions of game theory. All actors involved understood the dominant strategy and why it was the best strategy, and they chose not to play it. They chose not to end the world.
Dr. Thomas Schelling calls this “the event that didn’t occur,” and he devoted his Nobel prize lecture to the invalidation of von Neumann’s and game theory’s predictions by our 50+ years of applied experience.
What’s worse (for game theory) is that these results aren’t unique. When tested and observed in the laboratory and in the real world, human beings consistently buck its predictions. These anomalies are as common as grains of sand.
In ‘Altruistically Inclined?’ Dr. Alexander Field points out that the concept of reciprocity seems to be hard-wired into us12. In games of public good, the overwhelming majority of participants choose to help one another and their fellow human beings.
These anomalies represent a profound finding. And the more you think about them, the more you realize that we find humanity’s grace in game theory’s invalidation. Or, as Napoleon pointed out more than two hundred years ago,
The great mass of society are far from being depraved; for if a large majority were criminal or inclined to break the laws, where would the force or power be to prevent or constrain them? And herein is the real blessing of civilization, because this happy result has its origin in her bosom, growing out of her very nature.
However, the invalidation of game theory in this case, sadly, doesn’t mean that it’s strictly wrong.
In Part II, we’ll explore the one place where game theory does apply — within the midst and minds of those reaching for power.
The first few chapters are accessible at, https://www.economics.utoronto.ca/osborne/igt/.
As with most books that aren’t designed for auto-didacts, the book assume that an instructor/TA will help you solve some of the hardest problems that aren’t intuitively obvious. You can download the full solutions manual here, https://www.economics.utoronto.ca/osborne/igt/igtSolutions.pdf,
Accessible at, https://eudml.org/doc/159291 — English translation available at Google Books
Baym, G. and Hoddeson, L., 2004. Critical assembly. Cambridge: Cambridge Univ. Press, pp.130, 131. Accessible at, Google Books
Knuth, D.E., 1970. Von Neumann's first computer program. ACM Computing Surveys (CSUR), 2(4), pp.247-260.
Cohen, I., 2000. Howard Aiken. MIT Press Ltd, p.166. Accessible at, Google Books
The original report is accessible at, http://web.mit.edu/STS.035/www/PDFs/edvac.pdf , Wikipedia has an excellent summary of its contents.
Reed, G.A., 1986. “U.S. Defense Policy. U.S. Air Force Doctrine and Strategic Weapons Systems, 1958-1964: The Case of the Minuteman ICBM,” Ph.D. diss., Duke University
Note - As a Hungarian jew touched by the holocaust and the rise of fascism, von Neumann saw the rise of a nuclear USSR under Stalin as just as much an existential threat as Nazi Germany under Hitler.
Roberts, P., 2012. Cuban missile crisis. Santa Barbara: ABC-CLIO.
Summers, A., 2014. The arrogance of power. New York: Penguin Books. Relevant extract is accessible at, https://www.theguardian.com/weekend/story/0,3605,362958,00.html



Game theory is a science, not a philosophy. It can't be wrong. Only the math can be wrong. If people chose to pick an option which did not optimize their payoffs, then their payoffs were not properly represented.